D05: The wonderful world of sixths...

Here we express fractions as the sum of fractions whose denominators are powers of 6. This has parallels with the decimal fractions system, where the denominators are powers of 10. I'm not sure whether this will work successfully, but it is quite fun and might, at least, throw some light on division and multiplication algorithms!

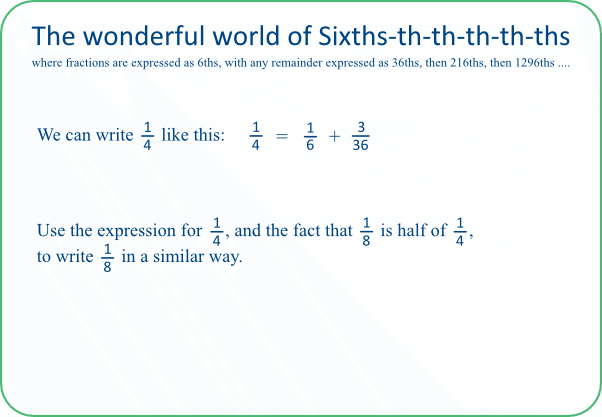
TASK 01A: Here we are shown how we could use division to construct an expression for ¼. Rather than simply divide 1 by 4, we divide 6/6 by 4 and then divide the remainder, expressed as 36ths, by 4. This time there is no remainder. If there were we would express it as 216ths and divide by 4 again!

For ⅛, we start by trying to divide 6/6 by 8. As 8 'doesn't go' we convert 6/6 into 36/36. Eight goes into 36 four times, with a remainder of 4, or rather 4/36 which is 24/216. Eight goes into 24 three times exactly. So we can write ⅛ = 4/36 + 3/216.

[It is quite nice to check the answer. It looks plausible: 4/36, or 1/9, is slightly less than 1/8 and 3/216 is small.  More precisely, 4/36 + 3/216 = 27/216 = (27×1)/(27×8) = 1/8.]

TASK 01B: This is a visual, or geometric, version of the first task.

The large square has been divided into 36 equal parts (or 36ths). We can see that the rectangle representing ⅛ covers 4½ of these parts, or four 36ths and three 216ths. So we can write ⅛ = 4/36 + 3/216.

TASK 01C: Back to using symbols. We can find the desired expression for ⅛ by dividing the given expression for ¼ by 2.

It is simpler to work from left to right. (Why?)
However, 2 'doesn't go' into 1 sixth, so convert it to 36ths, giving 9 in all. Two goes into 9 four times, with a remainder of one 36th or six 216ths. Two goes into 6 three times. So we can again write ⅛ = 4/36 + 3/216.

TASK 01D: This time, to get the desired expression, we multiply rather than divide the given expression for ¼, in this case by 3.

This time it is perhaps simpler to work from right to left. (Why?)
Three times 3/36 gives us 9/36 which we can think of as 1/6 and 3/36. Three times the given 1/6 gives us 3/6, making 4/6 in all. So we can write ¾ = 4/6 + 3/36.
[Again, it is worth finding ways to check this.]

You might want to extend the work. Some fractions, for example 1/27, are fairly easy to express in the desired way (1/36 + 2/216). Others, for example ⅕, lead to 'recurring' expressions. (Why?)