These tasks complement the tasks from the previous theme. This time, rather than finding the position of a fraction relative to the position of ⅓, we try to name the fraction, when we are given its position relative to ⅓ (and to 0).
It is likely that pupils will find these tasks to be easier than the corresponding tasks in themes 16 and 15, as they are slightly more structured.
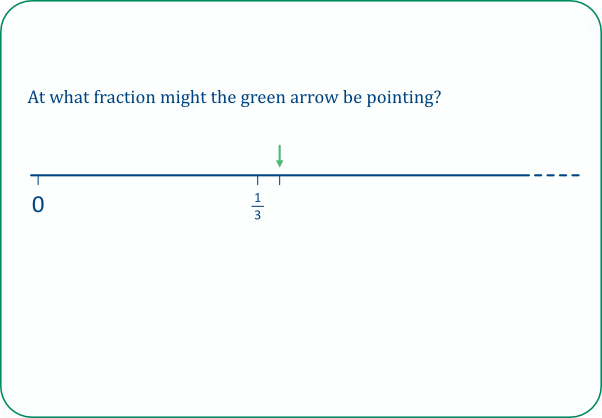
TASK 17A: Here the most plausible, simple answer is ½, as in Task 16A (though of course we can't be absolutely sure, even if we measure the intervals).
TASK 17B: Here the 'intended' fraction is ¼ as in Task 16B.
Again, one can't be sure that the arrow is pointing exactly to ¼, but it is the simplest 'plausible' answer. You might want to challenge pupils by asking,
If the fraction is not exactly ¼, what fraction might it be?
An interesting variation on this (and all the other tasks) is to provide some extra, equally spaced, marks. This won't necessarily make the task easier!
TASK 17C: The intended fraction here is ⅙, as in Task 16C. Easy!
TASK 17D: Here we were thinking of ²⁄₉. It will interesting to see whether pupils come up with other, plausible fractions; for example, ⅕ would be a good estimate!
TASK 17E: Here we were thinking of ¹¹⁄₃₀, as in Task 16E. However, one wouldn't expect pupils to come up with this precise fraction, unless they were asked (or they decided) to make very careful measurements.
