D15: Locating one third on a number line

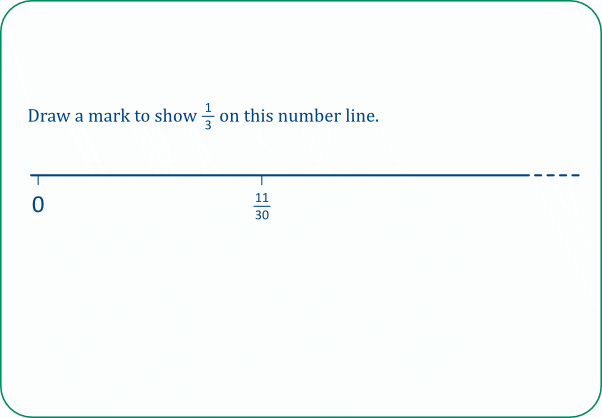
Here we have a simple family of tasks where we are given the position of one fraction on a number line and are asked to locate the position of the fraction ⅓. It is easy to vary the task, to make it more or less challenging.

TASK 15A: We start with the simplest of all fractions, ½, for the given fraction. The task, though, could pose quite a challenge to some pupils.

A general, or formal, way to solve the task is to convert the two fractions to fractions with the same (common) denominator, in this case to sixths: ⅓ is 2 sixths and ½ is 3 sixths, so ⅓ is two-thirds of the way along the interval from 0 to ½.

Some pupils might work in a more intuitive/visual way by making use of the fact that ½ lies midway between ⅓ and ⅔. This might lead to an image or sketch like the first line below, which can then be adjusted to make the intervals from 0 to ⅓ and ⅓ to ⅔ the same length (as in the second line below). You might want to see whether pupils can extend this method by asking them to locate ⅖, say, given the position of ½.

TASK 15B: The first task was quite challenging. This will be more challenging for many pupils, but perhaps not for those adept at using the notion of common denominator: the fractions can both be converted to 12ths. Pupils who were given Task 15A might spot that we can transform this task into Task 15A by using the position of ¼ to locate the position of ½.

A simple way to vary the task is to replace ¼ with other unit fractions such as ⅕ or ⅟₇. This doesn't alter the nature of the task substantially, other than that the fractions may seem less familiar to pupils and the fact that the practicalities of locating ⅓ are made more challenging by having to make use of more and smaller intervals. [Also the fraction ⅓ might go off the page....]

TASK 15C: This variant that is nice'n'easy. You might also want to try factions like ⅟₉ and ⅟₁₂ for the given fraction, though care would need to be taken in positioning the given fraction to ensure that the desired fraction, ⅓, doesn't go off the page.

TASK 15D: This is a bit more challenging again. You might also want to try fractions like ⁴⁄₉, ⅚ and ⁵⁄₁₂ for the given fraction.

TASK 15E: Here is a variant where we might be content with a qualitative answer: ⅓ is a 'tiny' bit to the left of the given fraction.

We can take this further by adopting a more analytical approach: ⅓ is ¹⁰⁄₃₀ and so is ¹⁰⁄₁₁ of the way along the interval from 0 to ¹¹⁄₃₀.

Here one might also want to try fractions like ⁹⁄₃₀ or ²¹⁄₆₀, or even ¹⁰⁄₃₁ or ¹⁰⁄₂₉, for the given fraction.